needed to explain observations and conserve energy
![]()
![]()
Conserved properties are very useful because they provide a way to successfully predict future events and measurements. While kinetic energy is conserved in elastic collisions, it is clearly not always conserved. Consider throwing an object straight upwards. Everyone knows the object will slow and for a moment stop its vertical motion. And then in what would seem to be a miracle if it were not so common observed, the object will gain kinetic energy as it starts to fall downward. And if the friction is trivial, the speed and kinetic energy at every height will be EXACTLY the same as they were at the same height while it traveled upward.
While one might propose that nature is so smart as to be able to create exactly the correct amounts of speed and kinetic energy, we prefer to assume that nature simply puts lost kinetic energy into storage in what we call potential energy. Such potential energy can be stored whenever an object is in a field of force which interacts with some property of the object. In the case of the thrown object, the force is gravity exerted on the object's mass. But energy is stored in electric fields when electric charges interact. And similar potential energy situations occur with all other force fields.
 William John Macquorn Rankine (b1820, d1872) was a Scottish physicist and railway engineer who with Rudolf Clausius and William Thomson (later called Lord Kelvin) contributed to develop thermodynamics. Rankine developed a theory based on circulating streams of elastic vortices to explain the steam engine and heat engines in general. His manuals of engineering science and practice were used for many decades after their publication in the 1850s and 1860s. Rankine published several hundred papers and notes on science and engineering topics. He defined and distinguished between actual energy which was lost in dynamic processes and potential energy by which it was replaced. He assumed the sum of these two energies to be constant. From 1854, he made wide use of his thermodynamic function which he later realized was identical to the entropy of Clausius (discussed in Expt. III-8).
William John Macquorn Rankine (b1820, d1872) was a Scottish physicist and railway engineer who with Rudolf Clausius and William Thomson (later called Lord Kelvin) contributed to develop thermodynamics. Rankine developed a theory based on circulating streams of elastic vortices to explain the steam engine and heat engines in general. His manuals of engineering science and practice were used for many decades after their publication in the 1850s and 1860s. Rankine published several hundred papers and notes on science and engineering topics. He defined and distinguished between actual energy which was lost in dynamic processes and potential energy by which it was replaced. He assumed the sum of these two energies to be constant. From 1854, he made wide use of his thermodynamic function which he later realized was identical to the entropy of Clausius (discussed in Expt. III-8).
A swing or weighted pendulum converts back and forth between kinetic and potential forms of energy. As energy stored in gravity pulls the swing downward, the taught, perpendicular support rope deflects the motion from downward to horizontal kinetic energy. As the swing continues the motion which is a portion of a circle raises the mass converting the energy back to gravitational potential energy. When friction is relatively low, a swing often rises nearly as high before momentarily stopping as it did on its previous swing.
For centuries people have tried to build perpetual motion machines hoping to improve the course of civilized life. The name perpetual motion machine is misleading because perpetual motion only requires either a frictionless situation (consider the planets and moons, and the motion of air molecules inside a thermos) or one in which friction is overcome by addition of replacement energy. What is of interest is an apparatus which would, because of its clever arrangement, generate sufficient motion so that it could do some outside work without slowing down or needing replacement energy.
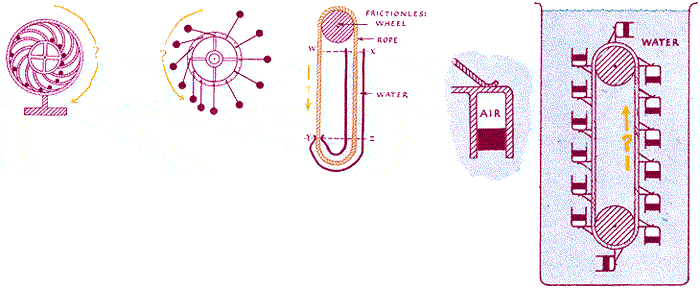
If we believe energy is conserved, then such an apparatus is impossible. Instead of suggesting that you start with your doubts and do your own experiments trying to build such an apparatus, rather try to find the flaws in the apparatus sketched below. This is not a matter that scientists are so convinced that energy is conserved that no perpetual motion apparatus could possibly work. There are many examples in the history of science where even the best scientists of the age have been wrong! But instead try to think though how each is intended to work, using your understanding of potential energy and other principles of physics to understand why they can't work. (The value in physics is not in memorizing vocabulary, names and formulas, but learning how to think clearly and apply what you learn about how our universe operates.)
The wheel on the left carries balls in curved compartments which roll downhill, giving added leverage to turn the wheel. The second wheel carries weights on spokes which pivot. The third is an endless loop of rope which floats in water. The seal is frictionless yet leakproof although that is not required for operation. The final apparatus on the right is an endless belt under water which carries cylindrical cups (one shown enlarged), each containing a heavy piston which can move with little friction controlling the compression of contained air. By displacing more or less water, the cups have different buoyancy on one side compared to the other.
Communicating technical information such as observations and findings is a skill used by scientists but useful for most others. If you need course credit, use your observations in your journal to construct a formal report.
Universally Conserved PropertiesThe concept that there are conserved properties has been of upmost importance to the develop our understanding of the universe. Conservation of mass, momentum, angular momentum, energy, electric charge, and others provide immensely valuable tools for understanding the world in which we live, predicting what events will happen, and modifying aspects of our world to better serve our best interests.Non-conserved Aspects of the UniverseBut there is considerable value in also realizing that there are other aspects of our universe which are not conserved. None of our individual lives are conserved. Love is not conserved. Beauty is not conserved. So unlike gold or gems which are prized as possessions, the beauty of a flower or a sunset can't be hoarded away. Those aspects of the universe are best shared, enjoyed, and celebrated when they are present and that is possible. There is no value in hiding our love, concern and caring for another person since those are opportunities which once past, are gone. Our lives are not entities which can be stored away, but once lived, we cannot go back in time and live differently. |
![]()
to next experiment
to e-Physics menu
to site menu