One of the properties of our universe that makes life as we know it manageable is that while some changes occur, many aspects do not change. These constants, and those aspects which periodically repeat (such as days and years), once understood, provide us power to control our lives and use our environment for life support. One of the more useful is what is now called mass, a term formerly used interchangeably with weight. We sense greater masses as heavier when we try to lift or accelerate an object.
For millennia humans have used weight as a commercial measure of materials because it is consistent and reliable. Its measure only changes when material is lost or gained. We say weight is conserved. For example it is universally accepted that the cost of twice the weight of, say, bananas should be twice as much. But it is equally useful to measure the weight of a substance by difference. For example the gravel in a truck load is determined by subtracting the net weight of the truck from the loaded weight. Chemists often determine the amount of material synthesized by summing the mass of ingredients. And particle physicists determine undetected electrically neutral elementary particles by the missing mass after summing the masses of all electrically charged particles.
As alchemy developed as the new millennia developed 2000 years ago, it seemed apparent that it was possible to change a substance with with one set or properties to another substance. Earthy elements could be appropriately treated with secret reagents creating a more valuable metal. But the processes seemed difficult, perhaps depending on using precisely the correct proportions of each material. Over many centuries the commercial use of weight for measuring materials became a more and more crucial practice for alchemy. The practice of carefully weighing reactants and products finally came to a head when a number of inconsistencies in then current alchemy theory were uncovered, leading to a scientific revolution. A group led by French amateur alchemist Antoine Lavoisier (b1743, d1794, shown at right with partner and wife Marie→) developed alternative chemistry theory based on weight. The concept of chemical element was redefined to be a substance separated and refined as much as possible. Weight became the crucial tool to determine whether a reaction added or removed materials.
The concepts of weight and mass have been tangled since ancient times. When commerce first began comparing quantities of goods, one way to judge equivalence was to heft each sample, using exertion of muscle force to lift and determine what is now called weight. But when equal-arm balances were used to provide a more accurate comparison, the effect of gravity cancelled from the comparisons leaving mass as the property being compared. For the purpose of commerce, weight and mass were effectively equivalent. For most human activities, there continues to be no compelling need to distinguish! But to continue to use the terms interchangeably when the difference is know is sloppy communication and promotes continued confusion.
Ancient peoples viewed the earth in the center of the universe and presumed all heavenly bodies naturally moved around the earth. The ancient Greeks saw no need for any other cause for heavenly motions. But after Nicholas Copernicus (b1473, d1543) suggested that the Earth might be a planet just like several other heavenly bodies, Johann Kepler (b1571, d1630) and others began to envision there might be some force needed such as magnetism or the gravity which caused the fall of objects on earth. Asked by Halley what would be the curve described by the planets on the supposition that gravity diminished as the square of the distance (r), Isaac Newton (b1643, d1727, ←portrait at left) immediately replied, an ellipseand claimed to have derived Kepler's three laws of planetary motion. When Halley asked to see the derivation, Newton was unable to find the papers. It took Newton 17 or 18 months of constant work to draft Mathematical Principles of Nature Philosophy describing the laws of motion and proposing a law of universal gravitation
Fgravity ∝ m1 m2 / r2
Newton used the term mass to both describe gravitational mass, m, that property which is involved in the creation of the gravity, as well as inertial mass, the property of objects which resists any change in their motions. Because gravitational mass and inertial mass mysteriously appear identical in all situations, we simply use the term mass to represent both. However Newton designated weight to represent that gravitational force, Fgravity, between masses, such as the pull of an apple by the Earth. This distinction between the concepts of weight and mass has remained clouded by confusion since the distinction has not been of importance to most people. But for physics, chemistry and many other activities the distinction is important. (In at least once case, a new Canadian passenger jet aircraft ran out of fuel in the air, mid-flight, when the amount of loaded fuel had been measured in units of pounds [weight] instead of the desired kilograms [mass].)While a distinction between weight and the various forms of mass have been considered unimportant by many people, the apparent conservation of these related properties has been crucially important! It was precisely because the weight of an object didn't seem to change over time and its equivalence to a similar object remained unchanged, that weight was taken as a valuable measure for commerce. But weight is not always conserved. If you precisely weigh an object in a valley and on a mountain top, there will be small weight difference. And if the object is transported to the moon, its weight will be much less there. It is because precisely measured mass does not even exhibit these changes that scientists distinguish and prefer to measure the mass of objects.
As a result of the actual use of mass in commerce, in alchemy and later chemistry, and in physics (despite the name confusion), mass became the first of a growing list of conserved properties which play a crucial role in understanding the very nature of our universe. Understanding the value of mass will help to understand other conserved properties such as momentum, energy, baryon number and many similar properties.
One might have thought that since we have all experienced mass and gravity all our lives, we should certainly know all about mass and gravity. Didn't Newton's Principia provide the complete details? While Newton did provide the mathematical description of gravity (and Einstein added some details for extreme situations), we still do not completely understand the cause of mass. This became clearer when physicists figured out electricity, the much stronger nuclear force, and even weak nuclear interactions. But the summary of those forces, known as the Standard Model, has some inconsistencies unless there is more to our explanation of mass.
Many of the fundamental particles in the universe have set masses. But theorists suspect that mass is not actually a property of these particles. A mechanism for mass was postulated by British physicist Peter Higgs (b 1929 ←photo at left) in 1964 while walking the Scottish mountains, and returned to his lab declaring he had had his one big idea. His theory hypothesizes that a sort of lattice, now referred to as the Higgs field, fills the universe. This is a bit like an electromagnetic field, in that it affects particles that move through it. It is like a famous person moving with a throng of fans has inertia created by the surrounding crowd; it is hard for the famous person to start moving, or once in motion, change course or speed. Higgs suggested the same could be true for particles in a Higgs field: a particle moving through it creates a little bit of distortion and that provides mass to the particle depending on the amount of attraction by the Higgs field.
Just as particles can be created by high enough energy accelerations in other types of force fields, acceleration in the Higgs field could create the last remaining class of particle needed to complete the Standard Model: the particle named the Higgs boson. The lightest
Higgs particle is predicted to be nearly within the range that the most powerful of the world's particle accelerators might create. In August 2000, physicists working at CERN's saw traces of particles that might fit the right pattern, but the evidence was inconclusive and the CERN particle accelerator was scheduled to be shut down for a major upgrade. As this is being written, CERN is preparing to restart the search with a now more powerful accelerator. Many scientists are eagerly awaiting what they expect to be the discovery of the first Higgs boson!
Mass is typically measured with a balance which compares an unknown mass with standard masses. But mass can also be calculated by measuring acceleration with a known force or weight in a known gravitational field. Below is a diagram of a simple inertial balance constructed using a steel strip such as a hack saw blade used to cut iron. It needs to be firmly attached at one end so that the support does not move nor distract from the blade's motion. If the free end is displaced to the side then released, it will regularly oscillate back and forth. The oscillations may be slowed by attaching one or more masses to the free end.
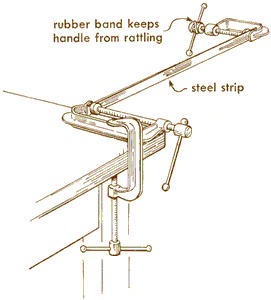
outfrom the support. Measure the average period, and construct a graph of period verses mass (say measured in units of the number of added masses). Be prepared to make apparatus modifications and repeat parts of the experiment as needed. For example, a stronger balance could be constructed by bolting two strips together at both ends with spacers keeping the two strips some distance apart but parallel.
weighunknown masses?
Communicating technical information such as observations and findings is a skill used by scientists but useful for most others. If you need course credit, use your observations in your journal to construct a formal report.
Finally, it might be interesting to ponder what life would be like if mass was not conserved. Life would be much more complicated if your own mass changed even regularly so that it would take different amounts of effort applied to your leg even to take a step!
to next experiment
to ie-Physics menu
to site menu
