![]()
What do each of the following graphs suggest about the motions they describe?
![]()
What do each of the following graphs suggest about the motions they describe?
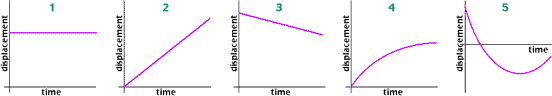
| Answers |
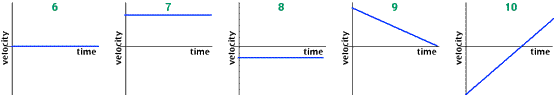
| Answers |
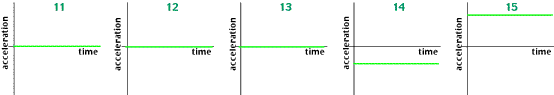
| Answers |
(Answers may be viewed by pointing to the Answers to the far right of each row of graphs.)
As noted in the third answer, the columns of graphs are related. The relation is provided by the definitions of the properties.
Recall that velocity: v ≡ Δd / Δt
and that acceleration: a ≡ Δv / Δt.
So going from a displacement graph, to a velocity graph, to an acceleration graph takes the changes (indicated by the Δ) in the former to produce the vertical value of the next graph. The steeper the slope (uphill to the right), the more positive the value. For example, a flat, zero slope velocity graph indicates a zero value acceleration.
And the converse is true going from acceleration to velocity to displacement graphs: A positive value indicates an uphill to right slope. The greater the value, the steeper the slope. For example, a slightly negative velocity indicates a slow displacement BACKWARDS (downwards on graph).
Note that progressing from displacement to velocity to acceleration loses information. If you are using a table of measured displacements (such as obtained in Experiment I-1), you will have fewer values for velocities and even fewer values for accelerations. If you wish to reconstruct a velocity graph from acceleration you must find the initial velocity elsewhere. And if you wish to reconstruct displacement data from velocity, you need to supply the missing initial displacement from the origin.
vfinal = a x Δt + vinitial
dfinal = v x Δt + dinitial
(There equations are obtained from the definitions by recalling that Δv = vfinal — vinitial and Δd = dfinal — dinitial. The equations are then rearranged to solve for the final values.)